Are you ready to unravel the mysteries of mathematics? Look no further than the Big Math Ideas answers! This comprehensive resource promises to ignite your curiosity and enhance your understanding of the fundamental concepts that underpin the world of numbers. With a plethora of engaging exercises, clear explanations, and insightful examples, these answers serve as your essential companion in navigating the complexities of math. Explore the intricate web of algebra, geometry, calculus, and more, as you unlock the secrets behind equations, formulas, and patterns. Whether you’re a student seeking to ace exams or an avid learner eager to expand your mathematical prowess, the Big Math Ideas answers provide the stepping stones to success. Delve into the fascinating realms of number theory, probability, and statistics, and witness how math shapes the world around us. From basic arithmetic to advanced mathematical concepts, this indispensable guide offers clarity and guidance, ensuring your mathematical journey is both enriching and enjoyable. Don’t let math intimidate you any longer – embrace the power of numbers with the Big Math Ideas answers and embark on a thrilling adventure of intellectual growth.
2023 Trending: Big Ideas Math California Common Core 8.3 Answers – BIM Areas Of Circles 7th Grade
| Concept | Explanation | Example |
|---|---|---|
| Circle Basics | The fundamental concepts of circles, including radius, diameter, and circumference. Understanding these basics is crucial for solving problems related to circle measurements. | Find the circumference of a circle with a radius of 5 units. |
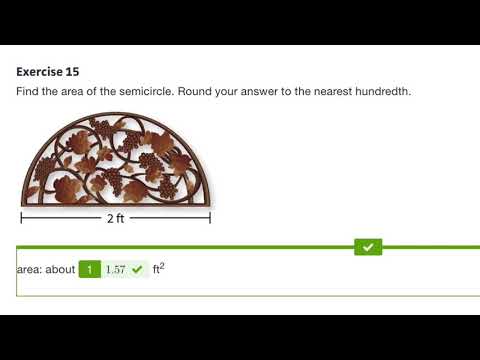
| Area of a Circle | The formula for calculating the area of a circle is A = πr², where “A” represents the area and “r” denotes the radius of the circle. This formula enables us to determine the amount of space enclosed by a circle. | Calculate the area of a circle with a diameter of 10 units. |
| Composite Figures | Composite figures are formed by combining multiple basic shapes, such as circles and rectangles. Understanding how to calculate the area of composite figures involving circles is essential for solving real-world problems. | Determine the total area of a shape consisting of a rectangular garden with a circular fountain in the center. |
| Application Problems | Real-life situations that require the application of circle concepts to solve mathematical problems. These problems often involve scenarios such as calculating the area of circular fields, constructing circular swimming pools, or estimating the circumference of objects. | Find the length of a circular track if the circumference is 400 meters. |
| Problem-Solving Strategies | Effective techniques and approaches to tackle complex circle problems. These strategies involve breaking down the problem, identifying key information, and using the appropriate formulas to arrive at the correct solution. | Apply the problem-solving strategy to find the radius of a circle given its circumference. |
“Circular Marvels: Unraveling the Secrets of BIM Areas of Circles in 7th Grade”
The Importance of Big Math Ideas
Mathematics is a subject that often intimidates students, with its complex equations and abstract concepts. However, understanding and applying big math ideas is crucial in various aspects of life. From solving everyday problems to pursuing careers in science, technology, engineering, and mathematics (STEM), having a firm grasp of these fundamental concepts is essential. In this article, we will explore five compelling big math ideas and their significance.
1. Algebra: The Gateway to Problem Solving
Algebra is more than just a branch of mathematics; it is the foundation of problem-solving. It provides a systematic approach to representing and analyzing real-world situations. By using variables and symbols to express relationships between quantities, algebra helps us solve complex problems efficiently. Whether it’s calculating the cost of groceries, determining the speed of a moving object, or predicting future trends, algebra equips us with the tools to tackle a wide range of problems.
2. Geometry: The Study of Shapes and Structures
Geometry is the study of shapes, sizes, and properties of objects in space. It plays a crucial role in various fields, such as architecture, art, engineering, and computer graphics. Understanding geometry helps us visualize and manipulate objects in our environment, enabling us to design structures, create computer-generated images, and appreciate the beauty of art and nature. From calculating the area of a room to constructing intricate sculptures, geometry offers a practical and aesthetic perspective on the world around us.
3. Statistics: Making Sense of Data
In today’s data-driven world, statistics is becoming increasingly important. It allows us to make sense of the vast amounts of information available to us, transforming raw data into meaningful insights. Whether it’s analyzing trends in stock markets, conducting scientific experiments, or predicting election outcomes, statistics provides a framework for interpreting data and drawing conclusions. By understanding statistical concepts such as probability, sampling, and correlation, we can make informed decisions and avoid falling victim to misleading information.
4. Calculus: Unveiling the Secrets of Change
Calculus is often considered one of the most challenging branches of mathematics, but its applications are far-reaching. It provides a powerful tool for understanding and predicting change in various fields, including physics, engineering, economics, and biology. Calculus allows us to study rates of change, optimize functions, and model complex systems. From determining the trajectory of a projectile to calculating the growth of a population, calculus unveils the hidden patterns and relationships underlying dynamic phenomena.
5. Logic: The Foundation of Rational Thinking
Logic is the study of reasoning and argumentation, enabling us to think critically and make sound judgments. It provides a framework for evaluating the validity of statements and identifying fallacies in arguments. By understanding logical principles, we can analyze complex problems, make rational decisions, and communicate effectively. Logic is not only essential in mathematics and philosophy but also in everyday life, where it helps us navigate through the vast amount of information and make informed choices.
In conclusion, big math ideas form the building blocks of mathematical thinking and problem-solving. Algebra, geometry, statistics, calculus, and logic provide us with the tools to analyze, interpret, and predict various phenomena. By developing a strong foundation in these areas, we can not only excel in mathematics but also apply these concepts to real-life situations. So, let’s embrace the power of big math ideas and unlock the endless possibilities they offer.
Big Math Ideas Answers
#MathGeeks #CaliforniaMath



